Unit load method also referred to as method of virtual work was developed by John Bernoulli in 1717. It is used to determine the slope and deflection at a specified point of a beam, frame, or truss.
In this method a virtual unit load is applied at the point of deflection (δ) and in the direction of deflection, whereas a unit virtual moment is applied at the point of desired slope.
By applying the principle of work and energy we get
external virtual work = internal virtual work
External virtual work =1kN.δ
Therefore, δ = Internal virtual work
Based on internal virtual work for different types of loading the deflection formula is given as follows;
Flexural members
In case of flexural members (beam, frame) the total internal virtual work is obtained by integration.
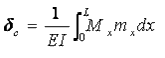
Where δ = deflection at point C
Mx = bending moment at x due to actual loading
mx = bending moment at x due to virtual unit load applied at point of deflection, C.
L = beam span
E = Modulus of elasticity of beam material
I = moment of inertia of beam section.
Note: In case of calculation of slope at a point we should apply a unit moment at the specified point of slope and write the equation for mx
See our solved Problem 7-3 for deflection of beam by unit load method.
Pin-jointed Truss
In case of truss the total internal virtual work is obtained by taking the algebraic sum
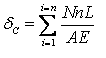
Where,
δC = deflection of joint C
N=axial force in a member due to real loading
n = axial force in the member due to virtual unit load applied at the joint C
L= length of member
A= area of cross-section of the member
E = modulus of elasticity of the member
Note: compressive forces are taken as negative whereas tensile forces are taken as positive.
See our solved Problem 7-5 for deflection of Truss by unit load method
For other problems please visit our Problem Solver
You can also use our Moment Distribution Calculator for solving indeterminate beams
Use our Fixed Beam Calculator to determine fixed-end moments for different loading cases.
You can visit the following solved examples on indeterminate beams and frames.
Excellent Calculators
Stress Transformation Calculator
Calculate Principal Stress, Maximum shear stress and the their planes
Calculator for Moving Load Analysis
To determine Absolute Max. B.M. due to moving loads.
Bending Moment Calculator
Calculate bending moment & shear force for simply supported beam
Moment of Inertia Calculator
Calculate moment of inertia of plane sections e.g. channel, angle, tee etc.
Reinforced Concrete Calculator
Calculate the strength of Reinforced concrete beam
Moment Distribution Calculator
Solving indeterminate beams
Deflection & Slope Calculator
Calculate deflection and slope of simply supported beam for many load cases
Fixed Beam Calculator
Calculation tool for beanding moment and shear force for Fixed Beam for many load cases
BM & SF Calculator for Cantilever
Calculate SF & BM for Cantilever
Deflection & Slope Calculator for Cantilever
For many load cases of Cantilever
Overhanging beam calculator
For SF & BM of many load cases of overhanging beam
More Links
Civil Engineering Quiz
Test your knowledge on different topics of Civil Engineering
Research Papers
Research Papers, Thesis and Dissertation
List of skyscrapers of the world
Containing Tall building worldwide
Forthcoming conferences
Containing List of civil engineering conferences, seminar and workshops
Profile of Civil Engineers
Get to know about distinguished Civil Engineers
Professional Societies
Worldwide Civil Engineers Professional Societies
Keep visiting for getting updated or Join our mailing list
Search our website for more...
PleaseTell your Friends about us if you find our website useful
Other Useful Links
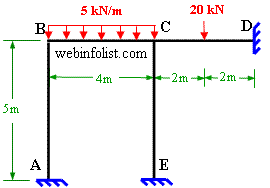 Problem
8-2New
Solution of Indeterminate frame by moment distribution method
Problem
8-2New
Solution of Indeterminate frame by moment distribution method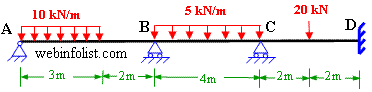 Problem
8-1New
Solution of Indeterminate beam by moment distribution method
Problem
8-1New
Solution of Indeterminate beam by moment distribution method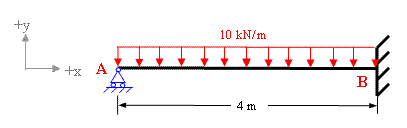 Problem 7-1 Solution of Indeterminate Structure - Propped cantilever
Problem 7-1 Solution of Indeterminate Structure - Propped cantilever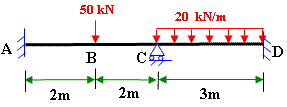 Problem 7-4 Solution of Indeterminate Structure by
slope-deflection equations
Problem 7-4 Solution of Indeterminate Structure by
slope-deflection equations