Problem 9-3
Compute the nominal flexural strength Mn of the reinforced concrete rectangular section given below in figure 9-3(a). Take fc′ = 5 ksi, beam width b = 14 in., effective depth d = 21 in. The beam has tension steel of As = 4-#10 bars placed in one layer fy = 60 ksi, and compression steel of As′ = 2-#7 bars, fs′ = 60 ksi, effective cover d′ of 2.5 in.
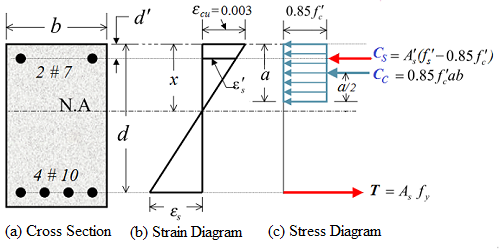
Figure 9-3
Solution:
The given section is doubly reinforced with steel in tension as well as compression zone of the section. The computation of nominal flexural strength Mn is based on the guidelines of ACI-318. The maximum value of usable strain at the extreme concrete fiber is assumed to be 0.003.
For fc′ grerater than 4000 psi the value of β1 is calculated as given below;
β1 = 0.85 - 0.05 {( fc′ -4000)/1000} = 0.8
Assume that the compression steel has yielded when the strength is reached (strain in concrete is 0.003).
Given that tension steel consists of 4 bars of #10 (dia 1.27 in.) .
Area of one bar of #10 = 1.27 in2.
As = 4-#10 bars. = 4 (1.27) = 5.08 in2.
Area of compression steel , As′ = 2-#7 = 2(0.6) = 1.2 in2.
The internal forces acting on the section shown in figure 9-3(c) are calculated as given below;
Cc = 0.85 fc′ ba = 0.85 (5) (14) a = 59.5 a
Cs = (fs′ - 0.85 fc′) As′ = (60 - 0.85*5) 1.2 = 66.9 kips
T = As fy = (5.08) (60) = 304.8 kips
Applying static equilibrium, we get Cc + Cs = T;
59.5 a + 66.9 = 304.8
therefore depth of stress block, a = (304.8- 66.9)/59.5 = 3.998 in
Depth of neutral axis x = a / β1 = 3.998/0.8 = 4.997 in
By straight line proportion (figure 9-3(b)) we can calculate the strain in compression steel when the extreme concrete fiber has a compressive strain of 0.003.
εs′ = ( x - d′ ) (0.003) / x = (4.997 - 2.5) (0.003) / 4.997 = 0.0015
yield strain of steel, εy = fy /Es = 60 / 29000 = 0.00207
εs′ is less than εy , this means that compression steel has not yielded before crushing of concrete. hence the assumption is not confirmed and therefore the calculated value of x is not valid.
In such cases when compression steel has not yielded we have to find the depth of neutral axis by considering the equilibrium of forces acting on the section;
Force in compression steel will be calculated on the basis of actual stress fs′ in compression rebar at the time of crushing of concrete.
fs′ = εs′ * Es ={( x - d′ ) (0.003) / x}*29000
Cs = (fs′ - 0.85 fc′) As′ =[{( x - 2.5 ) (0.003) / x}*29000 - 0.85 * 5] *1.2
Cc = 0.85 fc′ ba = 0.85 *5 *14 *(0.8*x)
T = As fy = 5.08*60 = 304.8 kip
Apply equilibrium of froces at the section;
Cc + Cs = T;
substituting the values of Cc, Cs, T in the above equation and simplifying we get;
47.6 x2 - 205.5 x - 261 = 0;
the above quadratic equation is solved to get the positive value of x;
therefore x = 5.34 in.
a = β1 x = 0.8 * 5.34 = 4.27 in.
εs′ = (5.34 - 2.5) (0.003) / 5.34 = 0.0016
fs′ = 0.0016*29000 = 46.4 ksi
Cc = = 0.85 *5 *14 *(4.27) = 254.065 kip
Cs = (46.4 - 0.85*5)(1.2) = 50.58 kip
Cc + Cs = 254.065 + 50.58 = 304.65 kip (equal to T; confirms calculation)
Nominal Flexural Strength
Mn = Cc (d - a/2) + Cs (d - d′ )
= 254.065 (21 - 4.27/2) + 50.58*(21 - 2.5) = 5728.66 in-kip
Mn = 477.39 ft-kip
Strain in the Tension Steel
Using strain diagram we can calculate the strain in tension steel
εs = 0.003(21 - 5.34) / 5.34 = 0.0088
The strain in the tension steel is more than yield strain and also greater than 0.005. Hence the section is tension-controlled. Therefore the strength reduction factor will be equal to 0.9
You can also use our reinforced concrete calculator to solve this problem or visit other solved examples on strength of Reinforced concrete beam Prob 9-1 and Prob 9-2
You can visit the following popular links of solved examples
You can also use following links of solved examples
Shear force and bending moment diagram for overhanging beamExcellent Calculators
Stress Transformation Calculator
Calculate Principal Stress, Maximum shear stress and the their planes
Calculator for Moving Load Analysis
To determine Absolute Max. B.M. due to moving loads.
Bending Moment Calculator
Calculate bending moment & shear force for simply supported beam
Moment of Inertia Calculator
Calculate moment of inertia of plane sections e.g. channel, angle, tee etc.
Reinforced Concrete Calculator
Calculate the strength of Reinforced concrete beam
Moment Distribution Calculator
Solving indeterminate beams
Deflection & Slope Calculator
Calculate deflection and slope of simply supported beam for many load cases
Fixed Beam Calculator
Calculation tool for beanding moment and shear force for Fixed Beam for many load cases
BM & SF Calculator for Cantilever
Calculate SF & BM for Cantilever
Deflection & Slope Calculator for Cantilever
For many load cases of Cantilever
Overhanging beam calculator
For SF & BM of many load cases of overhanging beam
More Links
Civil Engineering Quiz
Test your knowledge on different topics of Civil Engineering
Research Papers
Research Papers, Thesis and Dissertation
List of skyscrapers of the world
Containing Tall building worldwide
Forthcoming conferences
Containing List of civil engineering conferences, seminar and workshops
Profile of Civil Engineers
Get to know about distinguished Civil Engineers
Professional Societies
Worldwide Civil Engineers Professional Societies
Keep visiting for getting updated or Join our mailing list
Search our website for more...
PleaseTell your Friends about us if you find our website useful
Other Useful Links
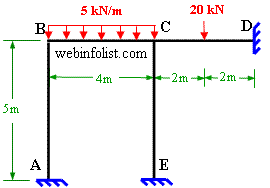 Problem
8-2New
Solution of Indeterminate frame by moment distribution method
Problem
8-2New
Solution of Indeterminate frame by moment distribution method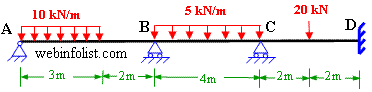 Problem
8-1New
Solution of Indeterminate beam by moment distribution method
Problem
8-1New
Solution of Indeterminate beam by moment distribution method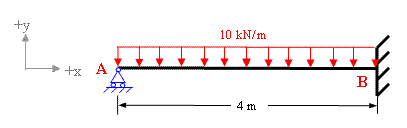 Problem 7-1 Solution of Indeterminate Structure - Propped cantilever
Problem 7-1 Solution of Indeterminate Structure - Propped cantilever Problem 7-2 Solution of Indeterminate Structure - Continuous Beam
Problem 7-2 Solution of Indeterminate Structure - Continuous Beam